... system.¹

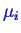For the partial derivative with respect to $X_i$ in Equation 20-3, there is a constraint between the variables $\sum X_i = 1$, that must be considered when taking the derivative of the molar free energy. Later, we will derive an important graphical construction for the determination of $\mu_i$ from curves of $\ensuremath{\overline{G}}$.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

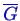... mixture²

That is a system of particles that occupy a neglible volume and are non-interacting. Each particle is identified with a chemical species. If the species are assumed to react, then the combinations of particles to form another vary according to the stoicheometric coefficients of a chemical reaction of the form $rR + qQ \ensuremath{\rightleftharpoons}bB + aA$

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...#tex2html_wrap_inline3301#
³

All the chemical species $i$ should use the same reference pressure so the subscript can be dropped. Whenever a subscript appears below, it will refer to a partial pressure, i.e., $\sum_i P_i = \ensuremath{{P}^{\mbox{total}}}$

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

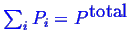
... gas.⁴

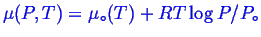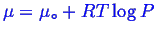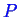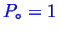An ideal single gas: $\mu(P,T)= \ensuremath{{\mu}_\circ}(T) + RT \log P/\ensuremath{{P}_\circ}$. Note, you will often see formulae like $\mu = \ensuremath{{\mu}_\circ} + RT \log P$, you know that $P$ cannot have units-so a convenient $\ensuremath{{P}_\circ} = 1$ has been picked.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.