Discussion

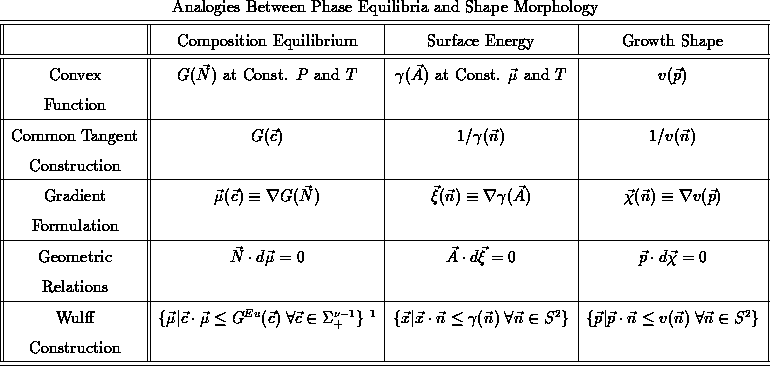
In this paper we have examined how the three topics to which Hubert Aaronson has contributes so much, phase equilibria, shape equilibria, and limiting shapes obtained with interface controlled growth, share a common mathematical basis, which is that in each case there are HD1 functions that are to be convexified. Because these topics have had separate developments, there are many methods that have been found useful in some but not all of the topics. These fall broadly into three areas as summarized in Table 1:

1. Those that operate on sub-manifolds of the HD1 function, such as , , and , and use such methods as finding its common tangents and curvatures to give phase and shape diagrams, as well as limits of metastability. Here the choice of metric plays a role in deciding which plot is to be convexified; and two choices for are contrasted below. The shape or -diagrams are simple analogs of phase diagrams with the missing orientations at edges and corners represented as two and multi-phase regions, and coexistent orientations represented by tie lines, triangles, etc.

2. Those that operate on the gradient of the HD1 function, the and plots and the characteristics to obtain shapes that have ready physical interpretation for and . Because of the Gibbs-Duhem relation the normals to any point on the plot gives its composition. The surfaces of the innermost parts of this plot represent equilibrium single phases and their composition ranges. Intersections to give edges and corners represent phases that are in equilibrium with one another. The missing orientations at edges and corners in this plot represent the composition gaps in the phase diagrams, and limiting orientations at these edges and corners.

   For the and plots the locally convex surfaces of the ``ears'' beyond these intersection represent metastable phases or surfaces; all other parts of the ears are separated from the metastable parts by spinodes and represent unstable phases or surfaces.

   There is no clear cut stability criterion for the characteristics; any characteristics can be stable at some time during shape evolution with arbitrary initial data. However the limiting shape of an outward growing crystal is the innermost plot, i.e. the plot without the ears.

3. Those that use a sub-manifold of the HD1 function in a graphical construction to obtain a shape that for and is the Wulff shape. For this is the shape with the lowest surface energy for the volume it contains; for this is the shape that for a given volume would add the least volume by further growth; it is also the limiting shape for outward growth. Such a construction can be done for , but the construction has to be modified because of the Manhattan metric for ; this is awkward. If we convert to a Euclimolar free energy, defined above, the unmodified Wulff construction works to give a -shape with equilibria alone.

The common mathematical basis has indeed made it possible to examine the analogies for all three topics and for all three basic methods. The differences in methods, such as common tangents on radial plots of versus , were often the result of the differences in the metrics in conventional use. Manhattan metrics work with the function; Euclidian metrics with the reciprocal. If we use a weighted metric for , such as energy/(unit area) projected along an axis, , the tangent constructions are done on this rather than its reciprocal. This metric is already in use for vicinal surfaces. Molar and molal free energies are convexified directly with equivalent results. The standard Wulff construction works with the Euclidian metric, and neither molar or molal free energies are easily used. But with the definition of a Euclimolar free energy we can directly create a graphically, without taking derivatives of or .

The analogies create many approaches for solving problems in all three topics. The advantages of having such flexibility in approach need to be explored. [44]

Phase diagram data are easy to obtain experimentally and can be obtained without knowing the free energy. Such data can be extrapolated. The topology of phase changes is guided by the phase rule; such phase changes appear on phase diagrams in standard formats. The same holds true for -diagrams; the orientation of smooth surfaces and the orientation gaps at edges and corners which develop at local equilibrium, i.e., without waiting for full shape equilibration or without measuring . From such data the -diagrams can be constructed and extrapolated, identifying surface ``phase changes'' that conform to a phase rule that is modified for crystal symmetry. We have in an example [54] exploited this interconversion between shapes and phase equilibria to analyze a complex series of phase changes in a ternary regular solution.

All the information that is in is not only in , but also in the plots; the free energies can be recovered from such a plot. The same interconversion holds for the chemical Wulff plots and the convexified free energies. These plots all display the same information, but in different formats. Furthermore some plots are more sensitive to errors in the data because differentiation or finding the point of tangency is involved. Which plot is best will be partially determined by the nature of the data; chemical potential data ought to go directly into constructing a -plot. Free energy plots show free energy and composition, but coexistent compositions must be determined by a tangent construction that is very sensitive to errors in the data and becomes increasingly difficult with increasing number of components. The Wulff shape is obtained without differentiating the free energy data; the shape requires taking a gradient of . These two plots should be congruent for the stable equilibria. They display phase coexistence clearly as corners and edges, compositions as normal directions, which implicitly requires differentiation, and free energy of a particular composition as the distance (in the appropriate metric) of the corresponding tangent plane from the origin.

The analogies are not perfect, as a few examples will show. The stability criteria are different for the kinetics. Curved surfaces can be part of the Wulff shape, and thus of an equilibrium shape. Only points in the -shape represent equilibria. Curved surfaces in this shape are ranges in the and in the compositions. A system that spans such a range is not in equilibrium and has real- space gradients of the chemical potential. While there is a clear analogy with edges and corners, there appear to be no chemical analogy to a triple junction of surfaces.