The Position of a Particle Executing a Random Walk
The Probability of Finding a Particle at a Position after a Random Walk
Treating the Concentration as Time-Dependent Probability Distribution
Relating the Self-Diffusivity to a Random Walk
A Puzzle: Why for a random walk is
![]() ?
?
The Successful Jump Frequency as an Activated Process
The treatment of diffusion as a statistical process permitted
a physical correspondence between the macroscopic diffusivity
and microscopic parameters for, average jump distance
![]() ,
jump correlation
,
jump correlation ![]() , and the average frequency
at which a jumper makes a finite jump
, and the average frequency
at which a jumper makes a finite jump ![]() .
.
In this lecture, the statistical evaluation of microscopic
process will be applied to the successful jump frequency ![]() .
A physical correspondence for
.
A physical correspondence for ![]() that is related to
microscopic processes of attempt or natural atomic vibration
frequency and the difference in energy between the potential energy
of site and the maximum value of the minimum potential energy (the
saddle energy) as the atom moves from one equilibrium site to the next.
that is related to
microscopic processes of attempt or natural atomic vibration
frequency and the difference in energy between the potential energy
of site and the maximum value of the minimum potential energy (the
saddle energy) as the atom moves from one equilibrium site to the next.
The result that will be obtain, that the frequency of successful hops,
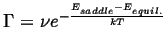 |
(14-1) |
Distribution of Energy among Particles
A fundamental result from statistical mechanics is that
for an ensemble of atoms at a fixed temperature ![]() , that
the energies of the atoms has a characteristic probability
distribution:
, that
the energies of the atoms has a characteristic probability
distribution:
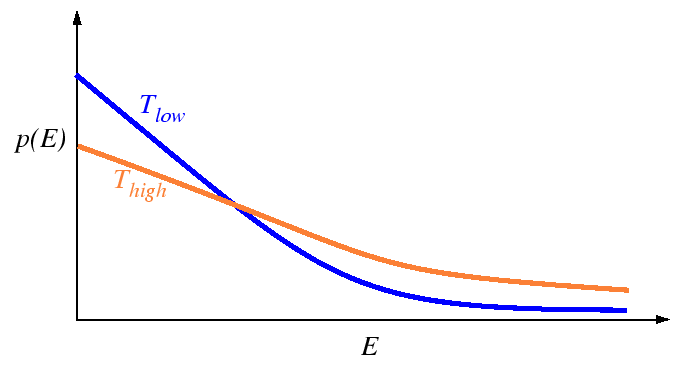 |
Below, the rate of successful jumps for simple models of activated processes will be derived. Each derivation will depend on the distribution of energies given above. It will be supposed that a single atom will assume all possible values of energy with probabilities given by the Boltzmann distribution over time (the ergodic assumption). In other words, the distribution is considered to apply to the atoms at a time scale that is rapid compared to the natural frequency of the atoms--no correlation is made for the loss (or gain) of energy as an atom hops from one equilibrium site to the next.
Activation Processes in Square Wells
Consider an ensemble of particles with distributed energies moving about on the following energy landscape:
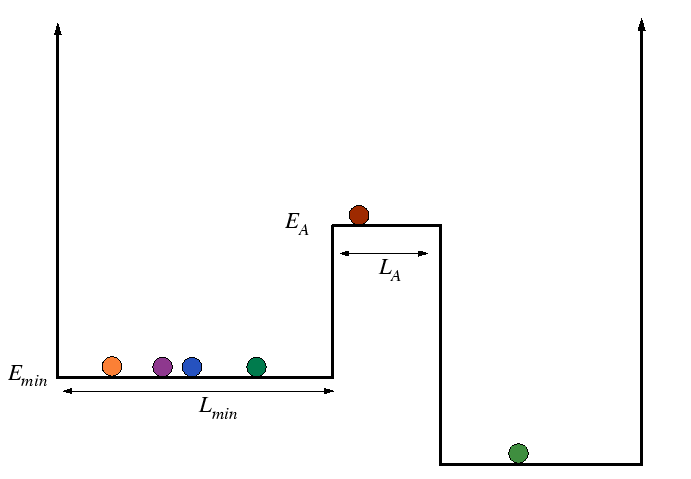 |
The characteristic time it takes a particle to cross the activated state is
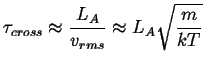 |
(14-2) |
The total rate, ![]() , that particles cross the barrier is
, that particles cross the barrier is
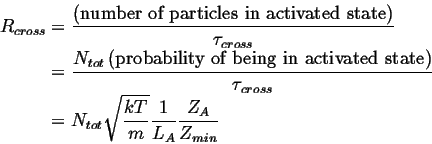 |
(14-3) |
The rate that single particle crosses, ![]() , is:
, is:
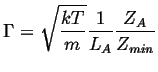 |
(14-4) |
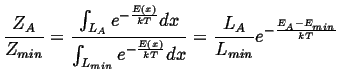 |
(14-5) |
Therefore,
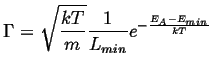 |
(14-6) |
The term that multiplies the Arrhenius factor (the 1/T exponential) is the characteristic time it takes a particle to make an attempt at the activated state.
Activation Processes in Harmonic Wells
Consider the following modification of the above simple case, the minima are treated as harmonic wells:
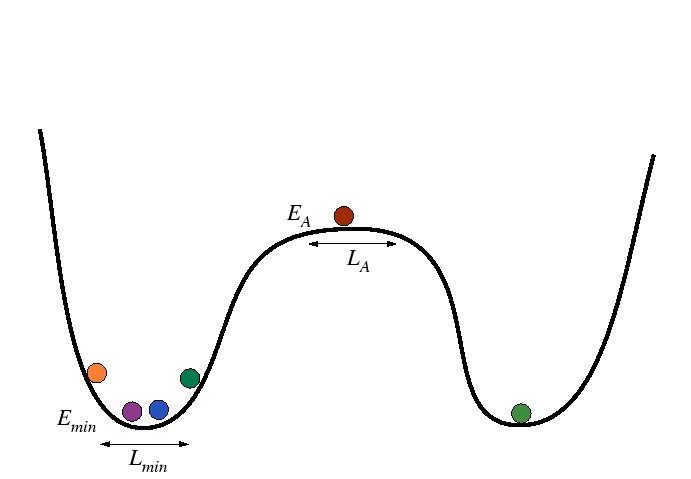 |
The minima can be approximated by
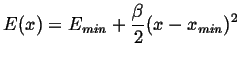 |
(14-7) |
The analysis is similar to the case of the square wells, but for the ratio of the partition functions:
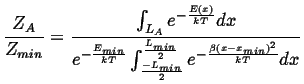 |
(14-8) |
Approximating,
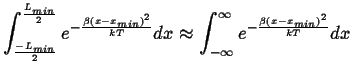 |
(14-9) |
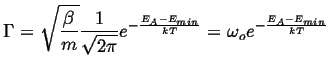 |
(14-10) |
Many-Body Theory of Activated Processes at Constant Pressure
In a real system, an atom or a vacancy does not make a
successful hop without affecting (or getting effected by) its
neighbors--all of the particles are vibrating and saddle point
energy is an oscillating target produced by the random vibrations
of all the atoms.
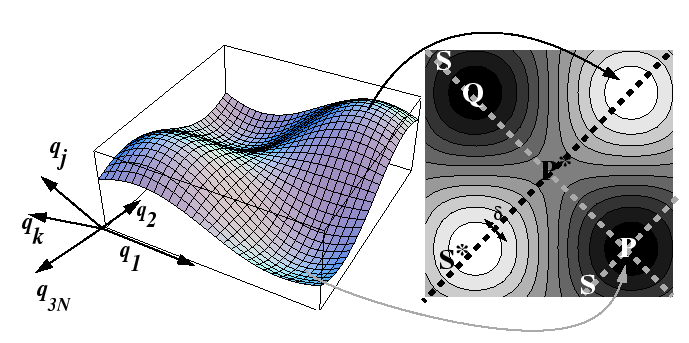
The
energy-surface that an atom, interstitial, or vacancy travels upon
is a complicated and changing surface.
If there are ![]() spherical particles, then there are
spherical particles, then there are ![]() -degrees
of freedom to this surface, but it will be assumed that the momentum
variables can be averaged out so that only a
-degrees
of freedom to this surface, but it will be assumed that the momentum
variables can be averaged out so that only a ![]() -dimensional
potential surface remains:
-dimensional
potential surface remains:
 |
The minima, or equilibrium values of momenta and positions, can be approximated by harmonic wells:
 |
(14-11) |
This is approximated by a one-dimensional problem by assuming that
all states during a hop lie on the surface ![]() in the figure.
in the figure.
Let the first coordinate be in the direction of the crossing
(parallel to ![]() ), then the average
(rms) momentum
), then the average
(rms) momentum ![]() in that direction is related to the an average rate
of attempts.
The result that was derived
for the harmonic potential can be re-used in this case:
in that direction is related to the an average rate
of attempts.
The result that was derived
for the harmonic potential can be re-used in this case:
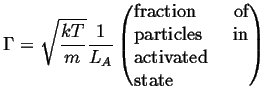 |
(14-12) |
However, in this case, the particle may have a different volume in the activated state compared to the equilibrium state:1
| (14-13) |
For the case where the volume may vary, but pressure is constant, the canonical constant pressure partition function must be used:
 |
(14-14) |
Therefore
![]() picks up an additional factor:
picks up an additional factor:
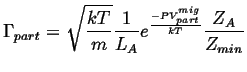 |
(14-15) |
It remains to evaluate the partition functions by summing
over all energies:
![]() .
The partition function is evaluated by passing to the classical limit
by dividing up the quantum phase space into cells of side-length equal
to Planck's constant,
.
The partition function is evaluated by passing to the classical limit
by dividing up the quantum phase space into cells of side-length equal
to Planck's constant, ![]() :
:
 |
Because of the uncertainty principle:
| (14-16) |
Each elementary volume,
![]() ,
in phase space must be considered to have degeneracy:
,
in phase space must be considered to have degeneracy:
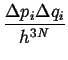 |
(14-17) |
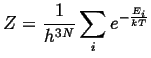 |
(14-18) |
In the classical limit,
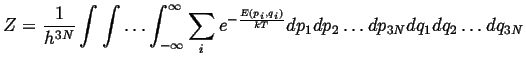 |
(14-19) |
 |
(14-20) |
Carrying out the same process for the activated state (which has one less degree of freedom) and adding the momentum near the activated state to the integral:
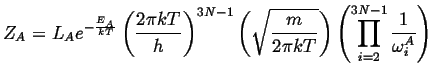 |
(14-21) |
The products over the vibrational modes can be related to the entropies of the states, i.e.,
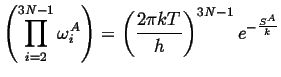 |
(14-22) |
Putting this all back into the expression for the rate of jumps,
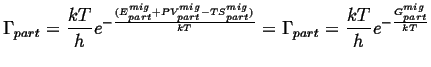 |
(14-23) |